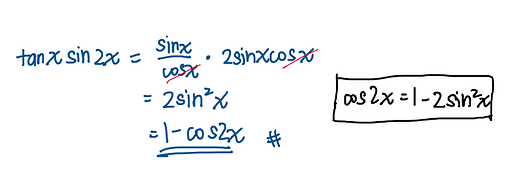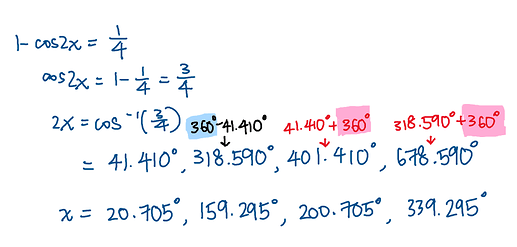Question
27 (a) Prove that \tan x \sin 2 x=1-\cos 2 x.
(b) Hence, solve the equation \tan x \sin 2 x=\frac{1}{4} for 0^{\circ} \leq x \leq 360^{\circ}.
(Ans : 20.705^{\circ}, 159.295^{\circ}, 200.705^{\circ}, 339.295^{\circ} )
Answer
Hi Farhaini,
For part (a), we try to change the expression into only sinx and cosx, here you notice the cosx cancels. Then we use the double angle formula for cos2x:
Hence our equation is proven.
In the actual exam, even if you can’t solve part (a), you can still solve part (b), because we always use the given result in (a). We add 360^{\circ} because 2x means two cycles.
Hope this helps! 
1 Like
Thank you very much! 


1 Like