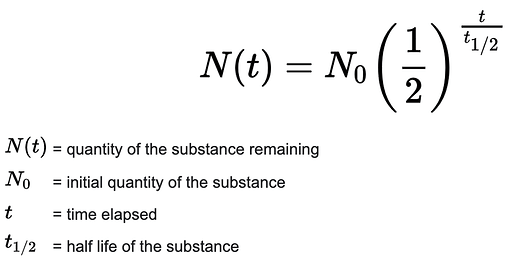[SPM Physics Radioactivity Solution] How to solve this question?

[Far]

[letian]

Hi Farhaini,

SPM Physics Radioactivity Question

49. A rate meter of a G-M tube recorded a background reading 40 counts per minute. When a radioactive element is put in front of the G-M tube, the rate meter reads 160 counts per minute. After 6 hours, the rate meter become 55 counts per minute.
    Meter kadar pada satu tiub G-M mencatatkan sinaran latar belakang 40 bilangan per minit. Apabila satu bahan radioaktif diletakkan di hadapan tiub G-M, meter kadar mencatatkan 160 bilangan per minit. Selepas 6 jam, bacaan meter kadar menjadi 55 bilangan per minit.

Determine the half life of the radioactive element.
Tentukan separuh hayat bagi bahan radioaktif itu.

A. 2 hours.
2 jam.
B. 4 hours.
4 jam.
C. 6 hours.
6 jam.
D. 12 hours.
12 jam.

SPM Physics Radioactivity Solution

We can begin by looking at the definition:

Real reading = Measured reading - Background reading
Half life = time taken for the radioactivity of an isotope to fall to half its initial value

Half life formula:

Applying the formula above:
Initial real reading = 160 - 40 = 120
Final real reading (after 6 hrs) = 55 - 40 = 15

Plugging in the values into the half life formula:
t = 6 hrs
N = Final reading = 15
No = Initial reading = 120

15 = 120 * (1/2)^(6 / half life)
15/120 = (1/2)^(6 / half life)

Adding log on both side to simplify the equation:
log(15/120) = log[(1/2)^(6 / half life)]

Logarithm rule: bring power down
log(15/120) = (6 / half life) * log(1/2)

Rearrange:
log(15/120) / log(1/2) = 6 / half life

3 = 6/half life

Final Answer:

half life = 6 / 3 = 2 hours

[Far]

I understand now Thank you very much!